A Pause in the Moment

I really like this time of the year, because the kids are very motivated and I know all of them well enough to pinpoint their strengths and weaknesses, and I have worked with them long enough to see a pattern in their learning and to see if they are growing in their efforts, responsibility, and habits of mind.

One thing I am trying this year (sort of organically) is to have all assignments be a dialogue with the students. They turn the assignments in, I look at them, give them back with written comments, and if the student's work isn't up to par, then the student knows that they need to re-do it (because I say it to them, leaving no assumptions). I think this back-and-forth dialogue is a more natural way of learning, and as a result I am a bit more lax on the deadlines. Unless a kid's work is more than a couple of weeks late, it matters more to me that it's done well than it is done absolutely on time. And it also helps to instill a culture of quality over quantity of work.

---------

We have an intern in our department this semester, and talking to her is bringing back all kinds of memories of my own first year of teaching! (All the hectic schedule, the stress, that feeling of momentary panic when you're standing in front of the room and kids won't listen, etc.) She's great though, definitely much better/firmer than I was as a first-year teacher. She's also multi-talented, totally certified to teach math, science, and German all at once. I'm still working on my one semi-professional qualification of teaching math...

Besides that, I think our department is finally, finally at a smooth-sailing pace. We've finally recovered from the shock of the start of the year, when everything was constantly backlogged. It only took us 4 months to get to this place. I can only say that the next year will be better, because I'll already know my way through the start of the year, and I can prepare for it better. Being a department chair is definitely not easy, but I think people have gradually warmed up to me in the last 4 months (and I have gradually gotten used to the responsibilities). By that, I mean now they don't bolt out the door at 4:30pm at the end of the meeting. They actually linger until we're done with business... which is a definite sign of something good, however small. I think I have a good relationship with everyone in the department, which really, really helps to smooth things over when issues arise. I cannot wait to see what the second half of the year will bring!

Late Autumn in Berlin

Time for a bit of general Berlin updates! (Math teachers who are not interested in this: Sorry, go ahead and move along in your GReader.)

October and November have been fairly busy months for us! First, the Jersey boys came to visit during Oktoberfest. It was a very rowdy good time (we had rented a house in the heart of Munich), and Geoff got all kinds of nostalgic thinking about how this might be the last time -- minus our wedding -- that they could have crazy, random experiences like this, since all the boys are nearing that age when they are thinking about settling down for good...

By the way, I don't know if you have ever been to Oktoberfest, but there they build these huge tents (like the one you see below) for only the month of that festival. It's incredibly hard to get in on Fridays and Saturday nights to one of the big tents, so we had to bribe the bouncers. Once you get in, the tent gets more and more crowded and crazier and crazier as the day rolls on...

While the boys were still here in Germany, we rented a Bier Bike, which is a mobile bar that you can pedal around Berlin while singing out loud and waving at people. We blasted Queen's Bicycle Race several times during the course of two hours on the bike, and we kept going afterwards to a hipster restaurant called White Trash, followed by karaoke until about 3am. It's very Berlin to have a bunch of random experiences all in one day, because this city is just organic and crazy!

Soon after the boys left, I heard via the grapevine that the Berlin Light Show was about to come to an end, and that it was cool to see the light show from the top of the Berlin Reichstag (Congress Hall). I didn't even know that you could make an appointment to visit the Reichstag at night! We had only been there once during the day. So, on a Sunday night in late October, we walked around Berlin to enjoy the light festivities on the famous monuments, and then went up the Reichstag.

Halloween was a bit quiet this year. On the actual day of Halloween itself, we had German class, so I went to class as normal and only wore my cat ears to be festive. My non-American classmates thought it was funny that I would celebrate this type of holiday still in costume. On the way back, I was impressed to see a holiday graffiti at the subway station, since it was quite a production and you know graffiti artists are seriously prosecuted by the subway officials. Later during the weekend, we went to a party where Geoff dressed up as the recycling goddess (he made a hula hoop skirt out of toilet paper rolls that we had collected for over 6 months, and also he made a busty bikini top out of cut-out juice containers), and I was dressed as eine Katz im Sack zu verkaufen, which means I was a cat inside a trash bag, with a for-sale sign taped to the bag. In German, "buying a cat in a bag" means to purchase something (such as a used car or an old building) without having seen it, so you don't really know how it's going to behave afterwards. In other words, I was playing off a German pun, but Germans don't really get why Americans would dress up as non-scary things for Halloween, so it was a fairly obscure costume...

Then, there was the Sparkle Army party this year! The Sparkle Army is an annual party at our favorite karaoke place, where if you dress up in sparkles then you can get in for free. We have some friends who have been going every year for about 5 or 6 years (since the inception of this idea). The slogan of the party is, amazingly, "More is more!" They really want to spread the word of sparkle. Last year we went to the party, but we were pretty last-minute about the preparations. This year, since I had coincidentally run into the Sparkle Army girls while they were shopping for supplies a weekend in advance, I went ahead and made advance preparations. We ended up bringing 20 of our people to the party. It was a blast!!

To give you just a small taste of why this party is awesome, here was actually some random guy (not one of our friends) with an absolutely awesome outfit. He even had slippers with glitter bulbs glued on, and there were stuffed animals sewn onto his pink tutu. Faaaaan-freakin-tastic!

whew. That's all the fall updates for now. December will get its own story, when it's time... My friend comes to visit this Friday, to enjoy Christmas markets in Berlin with us. I have planned already a Christmas market crawl -- in Santa Claus outfits (a la Santa Convention style, like you can find in some major cities in the States). I can't wait! Our cheap Santa costumes will get delivered on Wednesday, and we bought the most hilarious-looking ones with a funny-looking shoulder cape... Let the Christmas season begin!

Visualizing Complex Operations

I frequently feel sad that complex numbers are not part of the IB SL curriculum. During the time when I taught Algebra 2, it was always my favorite topic to connect algebra, geometry, and the history of numbers all at once.

Here is one cute activity I used to give to my students to illustrate the relationship between complex number operations and coordinate transformations. I recently gave it off-handedly to a student at our school, who was very intrigued by this, so I thought I'd share with those of you who still teach this topic. I think this activity is very eye-opening for the students and very visual, and it gives the various algebra operations a more concrete meaning / some motivation.

I vaguely remember that I had written about this a long time ago, but here I am posting it again since I cannot find the old post. (oops. Lost in the WWW, I guess.)

A Presentation with Optional Paths

I have been piecewise putting together a presentation I will be doing in the early spring at a conference called AGIS (for German international schools). I'm pretty nervous since it'll be the first time that I will be doing a presentation at a conference! My only previous presentation experiences were when I did a PD to my own department back in El Salvador, on utilizing web resources, and when I quickly presented something at PCMI 2011 for what I did with GeoGebra and unit circles.  Both of those were quite informal, I think, compared to speaking at a conference... So, I've given myself plenty of head start to think carefully about the content and presentation format for the talk in the spring. As a presentation newbie, I am nervous about not knowing who my audience will be. As a teacher who tends to lecture minimally in the classroom, I am also concerned about audience engagement as a whole when I speak to them one-sidedly for a stretch of time.

This presentation that I am preparing will be on math projects as a tool for self-differentiation. One thing I decided to do for this presentation is to not assume that all teachers are very experienced with projects, OR that they're completely inexperienced. So, my presentation will start by laying the foundation for why I think math projects are beneficial for students, just to establish a common ground with everyone. And then we go into pivot points, where I'll poll the audience quickly to find out which topics they would like me to spend the most amount of time speaking about. I was inspired by the idea that within powerpoint you can have hyperlinks to other parts of the powerpoint (eg. those Jeopardy-format powerpoints), so depending on their interest, we can click through only certain math projects to discuss them in more details and to spend some more time discussing general project structures, or to click through all of the projects and discuss actual content rather than format and framework of projects.

Here is the powerpoint I've pulled together so far. Please check it out -- using actual slideshow mode -- and give me feedback! Once you see the rounded boxes, you need to click inside the boxes in order to navigate forwards and backwards... (I used the boxes in order to avoid having ugly underlined hyperlinks everywhere.) Not all of the projects are mine, and I haven't filled all the slides in with pictures yet, but I am excited about the overall idea of a presentation that self-adjusts in real-time to the responses and vibes from the audience.

Addendum: I have revised my powerpoint to make it less verbose... Now I think it's 90 or 95% finished! Check it out and keep those suggestions coming! Thanks!!

Teaching Number Definitions Meaningfully

As a kid, I was never good with mathematical terms. I was always doodling in math class and only picked up vocabulary words osmotically (which also meant not so effectively). As a teacher, I have tried to make vocab instruction more meaningful for kids.

Recently, in Grade 8, we started talking about inequalities for the first time. I started off the discussion by asking kids to give me some examples of equalities, and we wrote them on the board. After a few minutes, we started to list examples of inequalities and I went over why in math, saying -5 is less than -2 is a bit more precise/less confusing than saying -5 is smaller than -2.

Then, I asked the kids to come up with some example numbers that can satisfy the inequality
x + 2 < 15 . The kids started to list numbers, and after a few minutes I asked them for what they wrote down, and in the context of this we discussed number types. I explained to them that I think generally, in life, when you want to brainstorm options in your head, you don't want to keep listing the same types of objects over and over again, because in doing so you are limiting your vision of what is possible. The examples that the first kid gave me were {1, 2, 3, ..... , 12} and the examples that the second kid gave me were {-1, -2, -3, -100}

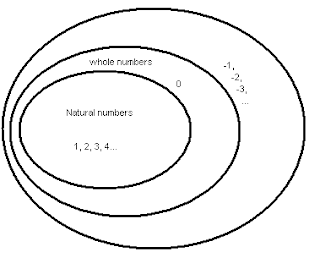
I drew on the board this diagram and said that "a long time ago, when cave people started counting sticks on the ground, they came up with numbers like 1, 2, 3, 4... These were called natural numbers.* Then eventually they came up with one more number to add to that set, and they called it whole numbers. Can you guess what that new number was?"

After kids enthusiastically guessed zero, they were starting to understand this diagram representation of a subset and were beginning to appreciate the historical development of numbers. Then, I added another layer of negative numbers and asked them what that is called when we started at some point to include/consider negative numbers as well.

They figured out that it was called integers. Great!

Then, I asked them what types of numbers they still know / have learned that we haven't named yet. They gave examples of decimals and fractions, and we added them to this picture.

In the end, we went back to the original topic at hand of finding example numbers that satisfy the inequality x + 2 < 15, and this time they were much better equipped to list a variety of examples and to discuss the full range of (non-discrete) solutions, which then led to the discussion of shading the solutions on the number line, and why we need the open circle rather than the closed circle sometimes.

I find this to be a more natural way to teach number types. Another time when I have done this was in teaching 9th-graders how to think of possible counterexamples that might disprove a math statement. If in their heads they are only considering a single type of example, then they're not being effective and thorough in their consideration of possibilities. As a kid, I would have appreciated this type of instruction of categorical types, followed immediately by application of its usefulness, and it would have probably helped me remember the names better. So, for me as a teacher, I always think it is important that I don't introduce the number definitions purely in isolation just because it's part of section 1.1 in the textbook. In the end, our teaching of these numerical categories should be explicitly supporting the kids' thinking, rather than just to add to the volume of disconnected rote knowledge in their heads...

*Note: By the way, I prefer this definition of natural numbers, even though I know that mathematicians don't all agree. Some refer to zero as part of the natural number set. 

A Day in the Life: Berlin Edition

 
Today was Friday. On Fridays, unofficially speaking, I have a full day with no free periods.

I got up around 6am and got ready. I knew it was going to be cold today, so I dressed extra warm with thermals and boots. Normally I eat breakfast, but today I was running late after responding to a Facebook message, and so I scrambled out the door at 6:32am with some dry cereal in a napkin to eat on the way to the train.

After a train and a bus, I arrived at school at 7:45am. Scrambled up to my classroom (on Floor 4), dropped off my stuff, and then hurried off to my morning Homeroom which began at 8am in another building.

After morning attendance with my Homeroom, I went to my Grade 9 class. Some of the kids who had opted to take the test today were pulled out of the room by the Student Support specialist. The rest of the kids were taking advantage of my extra review day for them. This was a double-period that lasted until 9:30 and seemed to fly. In the beginning of the period, I lost patience with one kid who was expecting me to hand him all of the answers, and I spoke to him impatiently and basically said that he was being lazy. I felt a little bad, but not too much. I told him that his effort was unacceptable and that I'm not one of those teachers who's going to say that it is OK what he's doing. The class was pretty quiet and really trying the problems after that. By the end, the kids were in good spirits because they felt like they have a grasp of the word problems dealing with midpoint and distance, even though they definitely still need to review quadratic factorization before next Wednesday's test... 

Then came morning break (a 15-minute recess), during which a boarding student came to speak with me about another new boarding student whom he is helping to tutor in math. We spoke about what the new student should be working on.

I grabbed my box of supplies (10 graphing calculators + board markers + lesson materials + 2 versions of textbooks) to head over to the other building where my next class was going to be. It was Grade 12. We went over homework from the previous day, which tied into the new Calculus stuff we were going to be doing -- area between two curves. I explained how this ties to the middle-school idea of finding an irregular shaded area using subtraction of total area minus smaller part/hole. The kids then practiced some skills in class and then copied down another practice quiz, due at the start of the next class. This double-period also went by fast. When class was over at 11:05, I looked up and was surprised that half the day was already over.

During lunch, 3 eleventh-graders came to see me for a re-quiz, and so did two seventh-graders. Before they left, I graded their quizzes on the spot, gave them feedback, and then pep-talked one girl who is really persistent despite her difficulties in Standard Level IB. I told her that as long as she keeps trying, I won't remove her from the class because I think it is possible for her to catch up, even though I know that her old math teacher didn't recommend her to go into Standard Level math. Another two Grade 9 twins tried to come get some help with their American curriculum (I have been teaching them the curriculum in pieces during lunch, since they're going back to the States after this year), but I told them that today's not a good day because I needed to prep for a class after lunch still. As usual, I just ate some bread which I had bought in the morning, for lunch. I always stay in my classroom during lunch, because kids tend to drop by at this time for re-quizzes, extra help, etc.

At the end of lunch, I headed over to teach PSHE, which is basically character education for my Homeroom kids. Today we were doing some bonding activities, because another Homeroom teacher was out, and some of her students were joining us. We did a get-to-know-you-better game involving asking provoking questions. The kids really enjoyed it, and they were sad when the period ended.

My own Homeroom kids then followed me to a different room for our Grade 8 math class. (I teach in many different rooms.) They turned in their lab report final drafts and we continued our discussion from yesterday of inequalities. They asked me interesting questions like, "Did it take people a long time to discover negative infinity?" And they also made up hand signals for infinity. I told them that they can start an infinity gang with their infinity gang signs, and after that every 5 minutes I'd see them flash the infinity sign.

After the Grade 8 math class, technically I should have two periods off at the end of the day, but those are the periods when I go in to support someone else's math class (unofficially). I work with a kid who moved to our school from a war-torn country, who is several years behind in math. I sit next to her and normally she works on solving simple one-variable linear equations while the rest of the class works on trigonometry. Today was the start of a new topic in Statistics, so she was following along the rest of the class and doing tallies. While she did tallies, since she said she didn't need any help, I just graded some recent trig projects for my Grade 11 students.

My colleague needed a bit of pep-talk after class, so I sat around to wait for her after school. We chatted a bit before I headed out to catch the bus. Today I left school early (at 3:30pm) because it's a Friday and also because I had a wedding dress fitting at 5pm in the opposite corner of Berlin.

The dress fitting went well! Afterwards, I grabbed dinner alone at my favorite Thai restaurant near home. Extra spicy. (It's my guilty pleasure to dine out alone. I do it every Friday so that I don't have to deal with talking to people at the end of the week, and plus it works out well that Geoff goes to play ball on Fridays.) I came home, updated the blog a bit, and then now I am thinking about how I should probably nap before my friend's Housewarmer tonight...

yawn.

Three-Variable Investigation... in Grade 8!

Even after teaching all of grades 7 through 12, I still LOVE teaching 8th-graders the best. (This is my 5th year teaching 8th-graders.) They have just accumulated enough algebra under their belts to be able to do rich explorations, and they are still so naturally curious about the world. They are like math ninjas, always ready to pull out their math skills to apply to the world at a short notice, and never intimidated by the look of a problem.

In the past couple of years, I realized that it's a real shame that we don't do modeling with three variables in middle-school math. It's a shame because it would be such a terrific tie in to the scientific process, to show how in mathematics you can also hold one variable constant while examining the effect of another variable on the output, and vice versa, and then in the end generalize the results into one grand conclusion. (I realized this because, looking at the past IB portfolios, this is a required skill for 11th- and 12th- graders. This was news to me as a person coming from the American curriculum.)

This year, I decided that I will try to remedy this gaping hole in our curriculum by exposing my 8th-graders to a new assignment. Take a look! They will be completing parts of this at home, then bringing it to class for discussions as a group. And then they will take more of it home to do. Eventually, when all kids feel comfortable with the process and the results, they will write it up like a modeling report. (We have already written one lab report this year based on Dan's awesome activity, and they found it very challenging / a great learning experience. In that lab, they had to learn how to define variables, collect data, determine the type of regression, perform regression, interpret results, make mathematical predictions, test their prediction, and then do error analysis. We followed it up with a very rigorous write-up process that included carefully critiqued rough drafts and a day spent on discussing how to create / insert graphs using GeoGebra and how to structure their writeup in a logical sequence.) Since I am a firm believer that kids learn more through writing about their understanding, the gears in my head are already turning to think about this next modeling assignment.

Anyway, I am VERRRRY excited about this three-variable assignment. Since the topic is already abstract, I kept the patterns linear to make it more accessible to all kids. But, I am very hopeful that it could turn out to be an awesome learning experience.

Addendum: For you new readers, this analogy is what I am going to use to kick off the introduction of 3-variable relationships in the real world.

Visualizing Order of Operations

This year my Grade 7s came in to my class with some missing prerequisite skills. Half of them started the year not knowing integers or order of operations or how to calculate simple fractions and percents, which are all supposed to be prerequisite knowledge for Grade 7 at our school. So, (although I did go back and fill in those gaps more or less,) teaching them algebra skills on top of this shaky foundation has been a new challenge.

At some point, I realized that it was difficult for these kids to look at a formula (in simple algebraic evaluation, for example) and to visualize what operations need to happen first, second, third, etc. They know the rules for PEMDAS, but it is just hard for them to always do it consistently. So, I came up with a trick of teaching kids to circle the operations in the given exercises in a layered manner, so that they can train their eyes to look for the operations, rather than to look at the equation from left to right.

Something like:

My special ed helper agrees that this is really helpful for them to visualize the rules. My only frustration is that they don't do this consistently because they still think they can just see it all in their heads, and in doing so they end up missing an extra negative sign here and there and throwing off the entire answer.

Anyway, I think the same trick can be used with certain types of equations to help kids see the layering and the process of peeling away the onion.

So, this is what I'll focus on for the next couple of weeks, to see if it can help solidify their foundation with this type of layered equation. (They're pretty OK with the ones with x's on both sides, since we had started off with doing balance visualization and crossing out shapes.) Basically, anything that can help them sink their teeth into abstract representation is worth a try for me. Any other ideas on how I can help these kids?

Ant on a Wheel

The hook for circular functions I had envisioned turned out to be pretty great, even better than I had imagined. The problem about the ant on the wheel was a hit, and really brought out some nice misconceptions from the kids. When I passed out the worksheet, I told them that they needed to estimate intelligently (not randomly guess) for the values in the table in #1, and that it should take a bit of time to complete if they were doing it correctly. They took their guesses, and most of them wrote things like

t = 0 --> h = 60
t = 0.5 --> h = 40
t = 1 --> h = 20
t = 1.5 --> h = 0
t = 2 --> h = 20
t = 2.5 --> h = 40
t = 3 --> h = 60
t = 3.5 --> h = 40
t = 4 --> h = 20
t = 4.5 --> h = 0


Then, when we discussed as a class, I drew a big wheel on the board and a vertical scale next to it to show height up to 60. I asked the kids where the ant was at 0 seconds, and everyone pointed to the top of the wheel, so I labeled it 0 sec. And then I asked them where the ant was at 3 seconds, and at 1.5 seconds, and we labeled those quickly as well since those were "obvious" to the kids. Then, less obviously, I asked them where on the wheel the ant was at 1 second and 2 seconds. To do this, they figured out that you have to divide the wheel up into thirds. And then we can further divide this wheel up to see where the ant is at 0.5, 2.5, 3.5, ... seconds.

By the end of our discussion, we got a diagram that looks something like this (on the board and also on their papers):

Each time, we referred horizontally over to the height scale that I had sketched on the board, and we estimated how high up the ant actually is. In doing so, the students noticed that they had to change the heights in their table. See below:

t = 0 --> h = 60
t = 0.5 --> h = 45
t = 1 --> h =15
t = 1.5 --> h = 0
t = 2 --> h =15
t = 2.5 --> h = 45
t = 3 --> h = 60
t = 3.5 --> h = 45
t = 4 --> h =15
t = 4.5 --> h = 0

One kid said, "But that doesn't make sense! I had divided it into equal parts before and that made sense." So, we had to discuss as a class that the ant is moving mostly vertically between certain parts of the wheel (ie. between 0.5 and 1 second, or between 2 and 2.5 seconds), and mostly horizontally between other parts of the wheel (ie. between 1 and 1.5 second, or from 1.5 to 2 seconds). Finally this kid is convinced that the height change is not a steady rate at all times.

So, with this consensus, I asked the kids to take out their graphing calcs and to create a scatterplot with this data (continuing the pattern all the way to 9 seconds, by 0.5 second increments, in order to reinforce their understanding of this circular pattern around the wheel). We looked at the graphs, and tada! It looks like a wave. Some kids were able to see it immediately, while others needed me to draw the scatterplot on the board and to connect it for them in order for them to see it.

Then, I asked them what type of function this was, and they were able to vaguely say sine or cosine (but they weren't sure which). We didn't get too far, but we started finding the equation of this function by hand for both sine and cosine functions and discussing the meaning of each part of the wave equation, which we will verify afterwards using the calculator's sine regression functionality. (Good time for the kids to practice all kinds of tech skills on the calculator, which they may or may not need for their internal assessment this year, depending on their internal assessment topics.)

Anyway, just thought I'd throw it out there. I didn't do super exploratory/introductory stuff this year in introducing waves, since the kids already have seen these equations the year before (in Grade 10), but I thought this lesson hook was really nice for re-introducing waves to them.

Addendum: I have decided that I'll be starting the next class by asking the kids where on the wheel the ant will likely raise two of his legs and say, "whee!" and then we draw it on the board on the wheel as well as on the wave graph, in order to connect this to rollercoasters and an itty bitty physics. On the other side, when the ant is rising rapidly, we can draw the ant hanging on barely, with two of his legs swinging in the wind.

Challenging Exponents/Log Problem

Fun/challenging problem from my colleague's test for his HL students (ouch!):

2(5^(x+1)) = 1 + 3/(5^x), solve for x in exact form "a + log(b)/log(5)", where a and b are both integers.

A student asked me after school for help with this, but I couldn't quickly figure it out on the spot. Afterwards, I thought over this and found it really fun to think of a variety of ways to try approaching this problem. (Too bad they mostly didn't work.) Over dinner, I thought of one way and it worked!

I am curious how you'd solve this. Please share!

Addendum: In hindsight, it's pretty foolish of me that I never looked at the graph even though I had suggested the graphical approach to that student. It's quite a simpler problem if you consider graphical approach as aid to the algebra. I was over-complicating things by doing it completely manually. (Still possible, but takes more work, obviously.) Silly silly me. These are the kinds of automatic-habit things I'd get better at, I guess, if I started coaching ISMTF competition teams....

Solo Performance and Remembering a Formula

I was doing a bit of sorting through old posts today, and I remembered that a couple of summers ago Sam Shah had asked me to blog about the cute activity I use to teach Quadratic Formula to my kids, since he says he cannot remember it otherwise.

Here it is: I teach them how to sing the Quadratic Formula song (to the tune of Pop! Goes the Weasel ), and then of course we practice in class how to apply the formula, and then for homework they need to go to a non-math teacher on campus and sing their song from memory. (To hold them accountable, I give them a half-sheet that says something like, "Dear Ms. Yang, I hereby certify that your student ___________ indeed came to me and sang from memory the following song to the tune of Pop! Goes the Weasel: X equals negative B, plus or minus square root.... [blah blah you know the rest], Signed, ____________")

It is very cute, because henceforth they sing every time they practice the formula, and they really do become experts at it before singing solo to a teacher who checks them off! And plus, some cool teachers even make them dance while singing. How very silly! I have kids who come back to me years after and still credit this assignment/song for them never forgetting that formula.

So, here you go, one of my sillier teaching "moves". I give you fair warning that after a few years you get really, really sick of hearing that tune... 

Intro to Waves...?

I am just going to try introducing waves like this this year in Grade 11. I hope it doesn't suck.

PS. They've "seen" sine and cosine functions before in Grade 10. Else I don't think you can introduce it this way and expect them to associate it with sine and cosine automagically. So I guess the proper title of this post would have been "Re-Intro to Waves...?"

100% Accountability

We had yesterday off from school. I had an epiphany during my day off that I am not instituting enough accountability and mental math drills in my Grade 7 class. So, today I remedied it with 80 minutes of a mini whiteboard lesson, and the result was really lovely.

We started off doing a laborious example: 2(x + 3), and I called on every single day-dreamer kid in the class to explain over and over again why this equals 2x + 6 (and as predicted, many of them could not say why even after their classmates had explained it 5 times). Once I was satisfied with our 100% accountability for listening in class, we proceeded on to do a very similar example, something like 4(x + 5). Everyone needed to do it on their whiteboards, and I asked them to all hold it up. 100% participation, no one can pass. We then practiced something like 5(x + 2) again just to make sure we all could do it. And then I started to call on one row at a time. When I called on the row, everyone in that row would stand up, and then I'd put up a question on the board. They'd be "put on the spot" to do it (individually on their boards without collaborating), and when they were all ready, they'd hold up their boards and their classmates would say whether they were correct.

This is a huge change from my normal mode of classroom learning, where I think I am too tolerant of mistakes, and so kids think that careless mistakes are totally ok even if you keep making them. This way, they're put under a bit of friendly peer pressure and they need to perform. --AND PLUS IT IS FUN!

We progressed our way to things like -(x + 3) or -8(3x + 5), and then -2(4x - 3) type of things, basic building blocks of algebra, until every group was consistently doing them correctly while standing for their turn.

Then, we moved on to doing two-step equations like 3x - 11 = 16. It turned out to be an excellent practice of their integer skills, and by the end of class, kids were comfortable finding fractional answers to two-step equations such as -4x + 5 = -2, and some can do it in their heads. Not bad for being week 2 of algebra for some of them (and having only recently learned about integers)!

So, I am liking this. Obviously it's not an everyday thing, but I think it adds a nice twist to my normal partnered work, and it really adds accountability for each student to be on the hook to do problems, correctly and consistently and independently. (I was very strict today about kids being silent and not talking during the exercises, and not asking their neighbors for help.) Truly, even my one student who normally gets no work done in class was really shining today and proudly displaying his work and volunteering to explain his answers to class. It was marvelous to see, and the special ed expert in the room was so impressed by the change in him.

So, go mini whiteboards on instituting 100% accountability!