I decided last night at around 10pm that I would try to get in to Berghain today. Berghain is the most famous techno club in Berlin -- and arguably the best club in the world -- and its fame is almost mythical. They are also one of the biggest gay-friendly clubs in Berlin. Their parties start on Friday nights and run all the way until Sunday evening, with no break in between. You can show up even at 9am on a Sunday morning to try to get in. The reputation of this party, which takes place in a run-down building in the middle of an abandoned lot, is that "they party like it's still the decadent 90s in New York City." If you are serious about entering, then you had better be dressed like a Berlin hipster, because the bouncers are very discerning. And, you could forget planning for a big group to all get in, because ironically you have to seem a bit "too cool to care" in order to get past the bouncers, and groups bigger than 2 or 3 almost never get in. For this reason, many Germans or people who have lived in Berlin for several years have not been to this world-famous club. (Some of my friends refuse to go, because they think it's ridiculous that you have to wait in line and then be arbitrarily rejected.)
Now, I am a math teacher, pretty much the opposite of a hipster. I can count on one hand the pieces of black clothing that I own, which are not brand-name skirts or dresses. Despite this, I wanted to at least attempt to see what Berghain is about before leaving Berlin. It's Pride Parade weekend, so I knew that getting in to Berghain would be tougher than ever, as it is one of the main after-party clubs. So, I got a full night's sleep and got up at 9am today (Sunday) to research what to wear in order to resemble a hipster. The websites are funny. They said that if you want to get in to Berghain, then you should make sure you "wear black and be skinny." And, "don't talk while waiting in line."
I threw together a pretty good hipster outfit, I think: Black long-sleeved t-shirt; a black-and-white textured skirt that I rolled up to make it shorter; a pair of ripped black tights (I had to take a pair of regular black tights and rip them by hand with scissors, since I don't keep tights once they get runs in them); a painted black leather bracelet; a dark fringe-looking necklace; and a deep red lipstick. I must have done an OK job with my hipster costume, because when I rolled on up to the door of the club, the bouncers automatically assumed that I had already entered previously and were asking to see my stamp for re-entry. When I said that I didn't have a stamp, they hesitated, looking into the club, and then said to me apologetically, "I'm sorry, lady... but not today."
--BUMMER!!!! I guess it must have still been very crowded inside, even though it was already 11am on a Sunday. (The parade was yesterday, and people flew in from all over Europe to party in Berlin. So, it's hardly a surprise that they were still partying a mere 24 hours later.) Well, since it's my last weekend in Berlin, I'll have to come back to Berlin and try to party at Berghain some other time! Now I have a whole rest of Sunday to hang out in my hipster costume. I think I'll go ahead and enjoy this -- being a hipster for a day. What do Berlin hipsters do on a Sunday? Do they go to brunch dressed in black and order eggs and cappuccinos, like everyone else??
PS. Maybe vegan brunch, over a box of cigarettes? (I don't smoke, unfortunately. But, if I did, I might be a more convincing hipster.....)
PPS. I found a Guide to Being a Hipster, thanks to Google. I will need to find ways to sneak into the conversation that the band has sold out, apparently.
Sunday, June 23, 2013
Monday, June 17, 2013
Sachen zu Tun im Berlin
If I had to make a list of top things that Berliners I know enjoy and recommend about the city, I'd say these are the top choices.
15. Go clothes-shopping in almost any Kiez, or neighborhood
14. Freiluftkino, or open-air cinema
13. Museums (Technical Museum, Berlin Historical Museum, DDR museum, bunker tours)
12. Go up the Reichstag to view the city and appreciate the glass dome architecture -- during the day or even at night (you can reserve times until about 11pm, I think)
11. East-side Gallery
10. Eat a Mustafa's doener kebab (totally worth the 1+ hour wait)
9. Biergartens in the park (Cafe Am Neuensee is my favorite! It's in Tiergarten.)
8. Ride your bike along the canal or from park to park
7. Party randomly until 7am (including going to a squatters flat, for a squatters' party)
6. Flea markets!
5. Kegel-bowling (traditional German bowling)
4. Go to a traditional German sauna (eg. Stadtbad Neukoelln)
3. Bierbike
2. Street festivals and open-air parties (such as Karnival der Kulturen or Mai Fest in the spring/summer time, and Christmas markets/Brandenburg Gate NYE party in the winter time)
1. (Sing at) Mauerpark's Bearpit Karaoke, in front of -- oh -- 3000 or so people at any given time!
Most of these I've done during my time in Berlin, but Geoff and I are running out of time to do the rest!! I have not had a chance to see an open-air movie here, and we've never been to a German sauna. We also haven't been to some of the recommended museums on the list. In the next week or two, this is my goal!! Chipping away at the bucket list like the best of them. Watch out!!!
15. Go clothes-shopping in almost any Kiez, or neighborhood
14. Freiluftkino, or open-air cinema
13. Museums (Technical Museum, Berlin Historical Museum, DDR museum, bunker tours)
12. Go up the Reichstag to view the city and appreciate the glass dome architecture -- during the day or even at night (you can reserve times until about 11pm, I think)
11. East-side Gallery
10. Eat a Mustafa's doener kebab (totally worth the 1+ hour wait)
9. Biergartens in the park (Cafe Am Neuensee is my favorite! It's in Tiergarten.)
8. Ride your bike along the canal or from park to park
7. Party randomly until 7am (including going to a squatters flat, for a squatters' party)
6. Flea markets!
5. Kegel-bowling (traditional German bowling)
4. Go to a traditional German sauna (eg. Stadtbad Neukoelln)
3. Bierbike
2. Street festivals and open-air parties (such as Karnival der Kulturen or Mai Fest in the spring/summer time, and Christmas markets/Brandenburg Gate NYE party in the winter time)
1. (Sing at) Mauerpark's Bearpit Karaoke, in front of -- oh -- 3000 or so people at any given time!
Most of these I've done during my time in Berlin, but Geoff and I are running out of time to do the rest!! I have not had a chance to see an open-air movie here, and we've never been to a German sauna. We also haven't been to some of the recommended museums on the list. In the next week or two, this is my goal!! Chipping away at the bucket list like the best of them. Watch out!!!
Friday, June 7, 2013
3-D Surface Area and Volume Projects: 2013 Edition
Here are the 3-D project photos from this year. I kept only the yellow tower and the red-and-yellow rocket from last year, so everything else on the shelves is new! As usual, they had to calculate volume, surface area, and draw 3-D designs and 2-D nets. Even though concavities were optional, many groups decided to build in concavities into their designs / calculations this year.
Afterwards, our tests were not easy, but the kids did quite well! In fact, the student who did the "star" project shown in the first picture (5 pyramids connected to a pentagonal prism, with a rectangular prism concavity) got 100% on her test, which made both her and me totally excited!!!!
Among other test problems, I offer you these two to try with your students. These problems integrate the flexible application of formulas with the necessary skill of visualization of volume and surface area. For an easy differentiation, you can replace one of the sides with x, and ask them to find an abstract formula for the SA or volume.
Calculate volume and surface area:
Calculate just surface area.
(Only standard circle equations for area and circumference are given.)
Intro to Lines the Visual Way
I wrote previously (apparently, a while ago) about how I introduce lines visually via patterns. After the end-of-year grades closed for Grade 7, I decided to pull together some materials and to have a go at this in the remaining weeks of the year, even though lines is really part of our Grade 8 curriculum. (My Grade 7s did very well on their end-of-year exam, that as a class there isn't much we need to go through and review again.) This intro to lines has been quite successful!! I incorporated the feedback from previous years, plus my own intuition of what works well as follow up, and came up with an introductory packet for the kids to work through.
Step 1: Writing equations of positive rates, from visual dot patterns.

Step 2: Drawing patterns from equations, in order to visualize the symbols within an existing equation.

Step 3: Writing equations with negative rate patterns, and then transitioning over to tables of values.
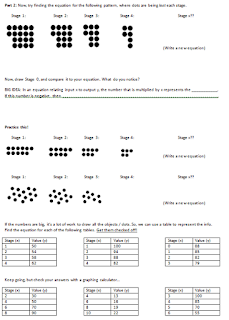
Step 4: Working with increasingly complex patterns with fractional rates (both positive and negative).

The result? They were hungry to get through it. They were looking for more. They were totally ready for the idea of the linear rate being "what happens, over how long it takes" by the end of this packet, and were able to write simple linear equations with no problem.
Subsequently, when I put up a table of values that had (6, 10), (9, 5), (12, 0) in it, many of the kids immediately were able to come up with the equation y = x(-5/3) + 20. Nice...! (Not all of them had really finished the packet at that point, since many of them were absent previously and were therefore a bit behind on the packet. So, this is really not bad. Even the kids who were a bit behind didn't have issues understanding why the equation would be true, when we discussed it as a class.)
So, here you go -- one of my last share-worthy materials of the year.
PS. This year, I didn't have them try to circle the missing/taken away dots in the negative rate cases. I just had them think about what is the value in stage 0. For example, if the pattern is
(1, 18), (2, 14), (3, 10), then they can first tell me that in stage 0, there are 22 dots. So, the equation is going to look like y = 22 + x(...). But, since we're not adding groups of 4, we don't want to write y = 22 + x(4). I just asked them, "How do we show that we're taking away 4 dots each time?" And the kids said, "Use negative 4!!" So, there, we write y = 22 + x(-4) together on the board, and they can re-arrange it into y = -4x + 22 if they'd like. Easy breezy. Then, in future work with tables of values, I just had to ask them, "Are we adding dots or taking away dots?" and they'd know to fix their signs on the rate.
PPS. And, I always read equations such as y = x(-5) + 22 out loud as, "We're starting with 22 in stage 0, and taking away x groups of 5." I think if we can approach it this way, kids will not likely confuse rate or slope with y-intercept, because they'd be thinking constantly about the meaning of the multiplication. More and more, I think that it is the language that we use to describe math that has a great impact on student understanding.
Step 1: Writing equations of positive rates, from visual dot patterns.

Step 2: Drawing patterns from equations, in order to visualize the symbols within an existing equation.

Step 3: Writing equations with negative rate patterns, and then transitioning over to tables of values.

Step 4: Working with increasingly complex patterns with fractional rates (both positive and negative).

The result? They were hungry to get through it. They were looking for more. They were totally ready for the idea of the linear rate being "what happens, over how long it takes" by the end of this packet, and were able to write simple linear equations with no problem.
Subsequently, when I put up a table of values that had (6, 10), (9, 5), (12, 0) in it, many of the kids immediately were able to come up with the equation y = x(-5/3) + 20. Nice...! (Not all of them had really finished the packet at that point, since many of them were absent previously and were therefore a bit behind on the packet. So, this is really not bad. Even the kids who were a bit behind didn't have issues understanding why the equation would be true, when we discussed it as a class.)
So, here you go -- one of my last share-worthy materials of the year.
PS. This year, I didn't have them try to circle the missing/taken away dots in the negative rate cases. I just had them think about what is the value in stage 0. For example, if the pattern is
(1, 18), (2, 14), (3, 10), then they can first tell me that in stage 0, there are 22 dots. So, the equation is going to look like y = 22 + x(...). But, since we're not adding groups of 4, we don't want to write y = 22 + x(4). I just asked them, "How do we show that we're taking away 4 dots each time?" And the kids said, "Use negative 4!!" So, there, we write y = 22 + x(-4) together on the board, and they can re-arrange it into y = -4x + 22 if they'd like. Easy breezy. Then, in future work with tables of values, I just had to ask them, "Are we adding dots or taking away dots?" and they'd know to fix their signs on the rate.
PPS. And, I always read equations such as y = x(-5) + 22 out loud as, "We're starting with 22 in stage 0, and taking away x groups of 5." I think if we can approach it this way, kids will not likely confuse rate or slope with y-intercept, because they'd be thinking constantly about the meaning of the multiplication. More and more, I think that it is the language that we use to describe math that has a great impact on student understanding.
Thursday, June 6, 2013
Teaching Tricks I Have Learned This Year
I'm always experimenting with different ways to explain things, and each year, I am happy if I can just find one or two "biggies" that really make a big difference for my students. Here are the gems I gathered this year. There are a lot of them this year, since I taught all the same classes as I did the year before. It has been a good year to refine my teaching techniques a bit...
1. Visualizing solving equations as "unwrapping the onion" to get back to x. The kids who practiced this were awesome at re-arranging equations in terms of variables, even if the equation looks like this and they're asked to solve for Q:
R = S + 3Q - 5W
T
2. Drilling equations of the form ax + b = c on mini whiteboards until every Grade 7 kid can do it in their sleep (including equations with fractional solutions). This really helped in the long run, because afterwards, when we moved on to much more complex equations, once they got down to an equation of this form, they could essentially do the rest while sleeping, and they wouldn't make any careless mistakes as their attention started to drift from the problem. It also helped them drill integer skills in algebra context, in the early stages of equation-solving.
Incidentally, I always read an equation like 5x = 3 as "5 copies of x is worth 3 in total. How much is each x worth?" I think this has helped my kids stay away from being confused about what to divide by what. If they can sound out the equation in their heads, the same way that I do, then it's not hard...
3. Always pronouncing fraction multiplication as "of" instead of "multiplied by", when reading an equation or expression out loud. For example, if the kids are simplifying (1/3)(x + 12), I say, "what is one-third of the quantity 'x plus 12'? Let's do it in steps. What is one-third of x? What is one-third of 12?" This helped to reinforce both the meaning of fractions and its connection to the multiplication operation.
4. Similarly, never let the kids get away with telling you that they don't know how to find a non-unit fraction, such as four-fifths of something. Always rephrase the question as, "What is one-fifth of ________? Then, how would you find four-fifths?" Push them, push them to do more in their heads. Don't let them think that fractions are harder than they are, or let them think that not knowing the meaning of fractions is OK.
5. Highlighting matching descriptions or units inside a proportion. Worked superbly to help kids set up proportions correct, consistently!
6. Teaching log by slowing down and focusing on its definition. This is a tried-and-true method for me this year; my students never had any weird issues at any point with logs this year, and I was able to repeat the same success with different students (some new transfers, some from other grades) at a later point. They were calm, independent, and their work all made sense from the start to finish during the entire unit. This had never happened to me before while teaching logs in any other way!!
7. Teaching sequences by making kids make a table of values (index vs. actual value) every single time, prior to setting up any equations. For some reason, this really slowed them down to thinking about what the word problem is giving them to work with, and they were consistently successful at tackling a variety of problems without getting confused.
8. Teaching Calculus by making kids sketch f'(x), f''(x), or f(x) graphs, given related graphs. They must do this consistently at the start of each class before moving on to work on anything else. The graphical understanding will underpin their entire algebraic understanding of Calculus, and help to bring everything together.
9. As soon as the kids differentiate a function via algebra, they must write down next to f(x) and f'(x) some word descriptions, such as "Height" for f(x) and "Gradient" for f'(x). This will build their independence in choosing the correct function to plug x-values into, and free them from having to ask you what to do at the next step of their analysis. Nag them while supervising/going over every problem, to write down these descriptions. Eventually, they won't need this anymore and they can visualize the descriptions in their heads. But, this builds their independence -- fast.
10. Repetitive quiz practice, on a complex topic, until you feel that it is quiz-worthy. This builds their confidence, while focusing their attention on a key skill, integrated with other skills they've seen before or that are nice to have.
I'm not done with the school year yet (still doing things that I'm pretty excited about, for the last few weeks of school), but I think that these are the little things that have made the most impact on my students' achievements/understanding this year. I hope that they will help you as well as they have helped me!!
1. Visualizing solving equations as "unwrapping the onion" to get back to x. The kids who practiced this were awesome at re-arranging equations in terms of variables, even if the equation looks like this and they're asked to solve for Q:
R = S + 3Q - 5W
T
2. Drilling equations of the form ax + b = c on mini whiteboards until every Grade 7 kid can do it in their sleep (including equations with fractional solutions). This really helped in the long run, because afterwards, when we moved on to much more complex equations, once they got down to an equation of this form, they could essentially do the rest while sleeping, and they wouldn't make any careless mistakes as their attention started to drift from the problem. It also helped them drill integer skills in algebra context, in the early stages of equation-solving.
Incidentally, I always read an equation like 5x = 3 as "5 copies of x is worth 3 in total. How much is each x worth?" I think this has helped my kids stay away from being confused about what to divide by what. If they can sound out the equation in their heads, the same way that I do, then it's not hard...
3. Always pronouncing fraction multiplication as "of" instead of "multiplied by", when reading an equation or expression out loud. For example, if the kids are simplifying (1/3)(x + 12), I say, "what is one-third of the quantity 'x plus 12'? Let's do it in steps. What is one-third of x? What is one-third of 12?" This helped to reinforce both the meaning of fractions and its connection to the multiplication operation.
4. Similarly, never let the kids get away with telling you that they don't know how to find a non-unit fraction, such as four-fifths of something. Always rephrase the question as, "What is one-fifth of ________? Then, how would you find four-fifths?" Push them, push them to do more in their heads. Don't let them think that fractions are harder than they are, or let them think that not knowing the meaning of fractions is OK.
5. Highlighting matching descriptions or units inside a proportion. Worked superbly to help kids set up proportions correct, consistently!
6. Teaching log by slowing down and focusing on its definition. This is a tried-and-true method for me this year; my students never had any weird issues at any point with logs this year, and I was able to repeat the same success with different students (some new transfers, some from other grades) at a later point. They were calm, independent, and their work all made sense from the start to finish during the entire unit. This had never happened to me before while teaching logs in any other way!!
7. Teaching sequences by making kids make a table of values (index vs. actual value) every single time, prior to setting up any equations. For some reason, this really slowed them down to thinking about what the word problem is giving them to work with, and they were consistently successful at tackling a variety of problems without getting confused.
8. Teaching Calculus by making kids sketch f'(x), f''(x), or f(x) graphs, given related graphs. They must do this consistently at the start of each class before moving on to work on anything else. The graphical understanding will underpin their entire algebraic understanding of Calculus, and help to bring everything together.
9. As soon as the kids differentiate a function via algebra, they must write down next to f(x) and f'(x) some word descriptions, such as "Height" for f(x) and "Gradient" for f'(x). This will build their independence in choosing the correct function to plug x-values into, and free them from having to ask you what to do at the next step of their analysis. Nag them while supervising/going over every problem, to write down these descriptions. Eventually, they won't need this anymore and they can visualize the descriptions in their heads. But, this builds their independence -- fast.
10. Repetitive quiz practice, on a complex topic, until you feel that it is quiz-worthy. This builds their confidence, while focusing their attention on a key skill, integrated with other skills they've seen before or that are nice to have.
I'm not done with the school year yet (still doing things that I'm pretty excited about, for the last few weeks of school), but I think that these are the little things that have made the most impact on my students' achievements/understanding this year. I hope that they will help you as well as they have helped me!!
Subscribe to:
Posts (Atom)





